www.michael-buhlmann.de

Funktionenbibliothek

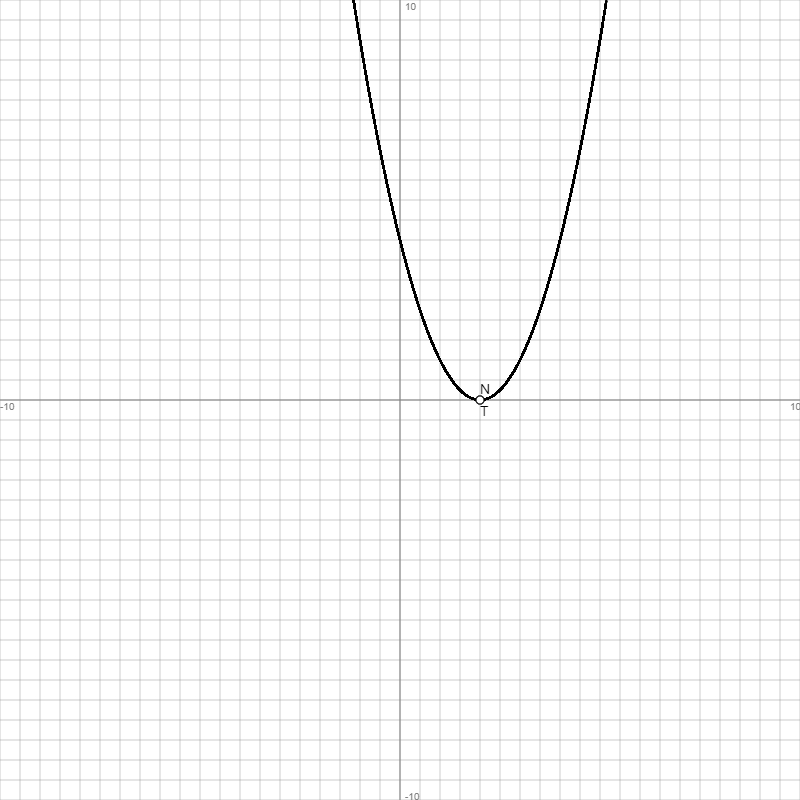
Funktion: f(x) = (x-2)², D_f = R, W_f = [0; +∞), entlang der x-Achse nach rechts verschobene Normalparabel (Scheitelform), Funktion achsensymmetrisch zur Senkrechten x = 2, x -> -∞: f(x) -> +∞, x -> +∞: f(x) -> +∞ ->

Wertetabelle:
x	f(x)	f'(x)	f''(x)	f'''(x)	Besondere Kurvenpunkte
-10	144	-24	2	0
-9.5	132.25	-23	2	0
-9	121	-22	2	0
-8.5	110.25	-21	2	0
-8	100	-20	2	0
-7.5	90.25	-19	2	0
-7	81	-18	2	0
-6.5	72.25	-17	2	0
-6	64	-16	2	0
-5.5	56.25	-15	2	0
-5	49	-14	2	0
-4.5	42.25	-13	2	0
-4	36	-12	2	0
-3.5	30.25	-11	2	0
-3	25	-10	2	0
-2.5	20.25	-9	2	0
-2	16	-8	2	0
-1.5	12.25	-7	2	0
-1	9	-6	2	0
-0.5	6.25	-5	2	0
0	4	-4	2	0	Schnittpunkt Sy(0|4)
0.5	2.25	-3	2	0
1	1	-2	2	0
1.5	0.25	-1	2	0
2	0	0	2	0	Nullstelle N(2|0) = Tiefpunkt T(2|0)
2.5	0.25	1	2	0
3	1	2	2	0
3.5	2.25	3	2	0
4	4	4	2	0
4.5	6.25	5	2	0
5	9	6	2	0
5.5	12.25	7	2	0
6	16	8	2	0
6.5	20.25	9	2	0
7	25	10	2	0
7.5	30.25	11	2	0
8	36	12	2	0
8.5	42.25	13	2	0
9	49	14	2	0
9.5	56.25	15	2	0
10	64	16	2	0
Graph:

Abkürzungen: D_f = (maximaler) Definitionsbereich, f(x) = Funktion, f'(x) = 1. Ableitung, f''(x) = 2. Ableitung, f'''(x) = 3. Ableitung, H = Hochpunkt, N = Nullstelle, P = Polstelle, R = reelle Zahlen, T = Tiefpunkt, W = Wendepunkt, W_S = Sattelpunkt, W_f = Wertebereich, {.} = ein-/mehrelementige Menge, [.; .] = abgeschlossenes Intervall, (.; .) = offenes Intervall, [.; .), (.; .] = halboffenes Intervall, ∞ = unendlich.

Bearbeiter: Michael Buhlmann

Zurück