www.michael-buhlmann.de
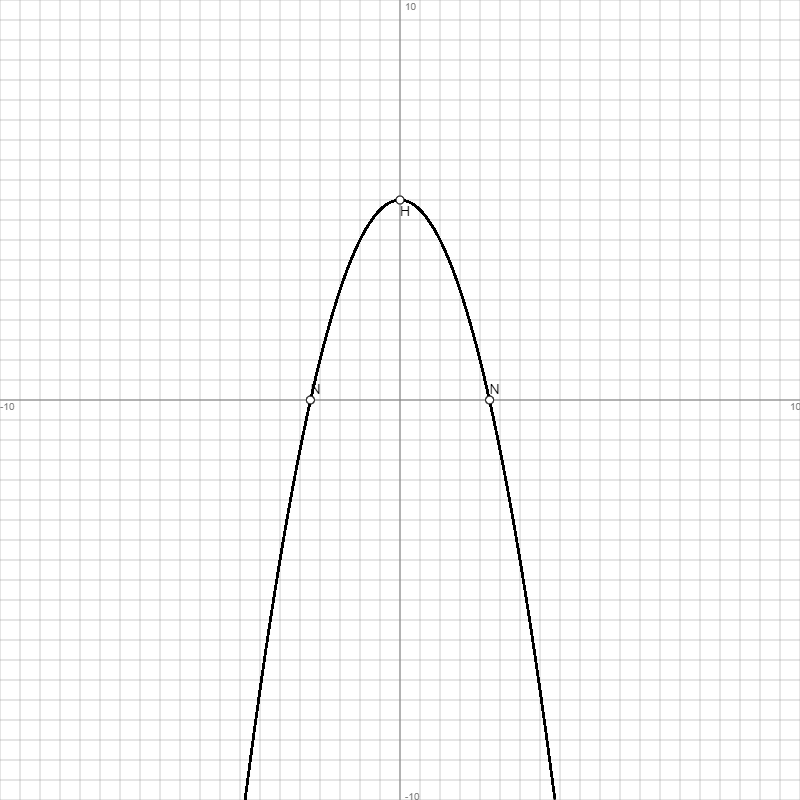
Funktion: f(x) = -x2 + 5, Df = R, Wf = (-∞; 5], nach unten geöffnete, entlang der y-Achse nach oben verschobene Normalparabel, Funktion achsensymmetrisch zur Senkrechten x = 0, x -> -∞: f(x) -> -∞, x -> +∞: f(x) -> -∞ ->
| Wertetabelle: | |||||
| x | f(x) | f'(x) | f''(x) | f'''(x) | Besondere Kurvenpunkte |
| -10 | -95 | 20 | -2 | 0 | |
| -9.5 | -85.25 | 19 | -2 | 0 | |
| -9 | -76 | 18 | -2 | 0 | |
| -8.5 | -67.25 | 17 | -2 | 0 | |
| -8 | -59 | 16 | -2 | 0 | |
| -7.5 | -51.25 | 15 | -2 | 0 | |
| -7 | -44 | 14 | -2 | 0 | |
| -6.5 | -37.25 | 13 | -2 | 0 | |
| -6 | -31 | 12 | -2 | 0 | |
| -5.5 | -25.25 | 11 | -2 | 0 | |
| -5 | -20 | 10 | -2 | 0 | |
| -4.5 | -15.25 | 9 | -2 | 0 | |
| -4 | -11 | 8 | -2 | 0 | |
| -3.5 | -7.25 | 7 | -2 | 0 | |
| -3 | -4 | 6 | -2 | 0 | |
| -2.5 | -1.25 | 5 | -2 | 0 | |
| -2.237 | 0 | 4.47 | -2 | 0 | Nullstelle N(-2.24|0) |
| -2 | 1 | 4 | -2 | 0 | |
| -1.5 | 2.75 | 3 | -2 | 0 | |
| -1 | 4 | 2 | -2 | 0 | |
| -0.5 | 4.75 | 1 | -2 | 0 | |
| 0 | 5 | 0 | -2 | 0 | Schnittpunkt Sy(0|5) = Hochpunkt H(0|5) |
| 0.5 | 4.75 | -1 | -2 | 0 | |
| 1 | 4 | -2 | -2 | 0 | |
| 1.5 | 2.75 | -3 | -2 | 0 | |
| 2 | 1 | -4 | -2 | 0 | |
| 2.236 | 0 | -4.47 | -2 | 0 | Nullstelle N(2.24|0) |
| 2.5 | -1.25 | -5 | -2 | 0 | |
| 3 | -4 | -6 | -2 | 0 | |
| 3.5 | -7.25 | -7 | -2 | 0 | |
| 4 | -11 | -8 | -2 | 0 | |
| 4.5 | -15.25 | -9 | -2 | 0 | |
| 5 | -20 | -10 | -2 | 0 | |
| 5.5 | -25.25 | -11 | -2 | 0 | |
| 6 | -31 | -12 | -2 | 0 | |
| 6.5 | -37.25 | -13 | -2 | 0 | |
| 7 | -44 | -14 | -2 | 0 | |
| 7.5 | -51.25 | -15 | -2 | 0 | |
| 8 | -59 | -16 | -2 | 0 | |
| 8.5 | -67.25 | -17 | -2 | 0 | |
| 9 | -76 | -18 | -2 | 0 | |
| 9.5 | -85.25 | -19 | -2 | 0 | |
| 10 | -95 | -20 | -2 | 0 | |
| Graph: | |||||
 | |||||
Abkürzungen: Df = (maximaler) Definitionsbereich, f(x) = Funktion, f'(x) = 1. Ableitung, f''(x) = 2. Ableitung, f'''(x) = 3. Ableitung, H = Hochpunkt, N = Nullstelle, P = Polstelle, R = reelle Zahlen, T = Tiefpunkt, W = Wendepunkt, WS = Sattelpunkt, Wf = Wertebereich, {.} = ein-/mehrelementige Menge, [.; .] = abgeschlossenes Intervall, (.; .) = offenes Intervall, [.; .), (.; .] = halboffenes Intervall, ∞ = unendlich.
Bearbeiter: Michael Buhlmann